How to Use the Chain Rule for Derivatives

Chain Rule

The chain rule is used to find the derivatives of composite functions like (x²+ 1)³, (sin 2x), (ln 5x), e^2x,and so on. If y = f(g(x)), then y' = f'(g(x)). g'(x). The chain rule states that the instantaneous rate of change of f relative to g relative to x helps us calculate the instantaneous rate of change of f relative to x. Let us learn more about the chain rule formula and the steps followed in finding the derivatives using the chain rule.

1.	What is Chain Rule?
2.	Chain Rule Formula And Proof
3.	Double Chain Rule
4.	Applications of The Chain Rule
5.	FAQs on Chain Rule

What is Chain Rule?

This chain rule is also known as the outside-inside rule or the composite function rule or function of a function rule. It is used only to find the derivatives of the composite functions.

The Theorem of Chain Rule: Let f be a real-valued function that is a composite of two functions g and h. i.e, f = g o h. Suppose u = h(x), where du/dx and dg/du exist, then this could be expressed as:

change in f/ change in x = change in g /change in u × change in u /change in x.

This is given as Leibniz notation in the form of an equation as df/dx = dg/du .du/dx.

Chain Rule Steps

Step 1: Identify The Chain Rule: The function must be a composite function, which means one function is nested over the other.
Step 2: Identify the inner function and the outer function.
Step 3: Find the derivative of the outer function, leaving the inner function.
Step 4: Find the derivative of the inner function.
Step 5: Multiply the results from step 4 and step 5.
Step 6: Simplify the chain rule derivative.

For example: Consider a function: g(x) = ln(sin x)

g is a composite function. So apply the chain rule.
sin x is the inner function and ln(x) is the outer function.
The derivative of the outer function is 1/sin x.
The derivative of the inner function is cos x.
Finally g'(x) = derivative of the outside function, leaving the inside alone × the derivative of the inside function = 1/sin x × cos x
On simplifying we get, cos x/sin x = cot x

Chain Rule Formula and Proof

There are two forms of chain rule formula as shown below.

Chain Rule Formula 1:

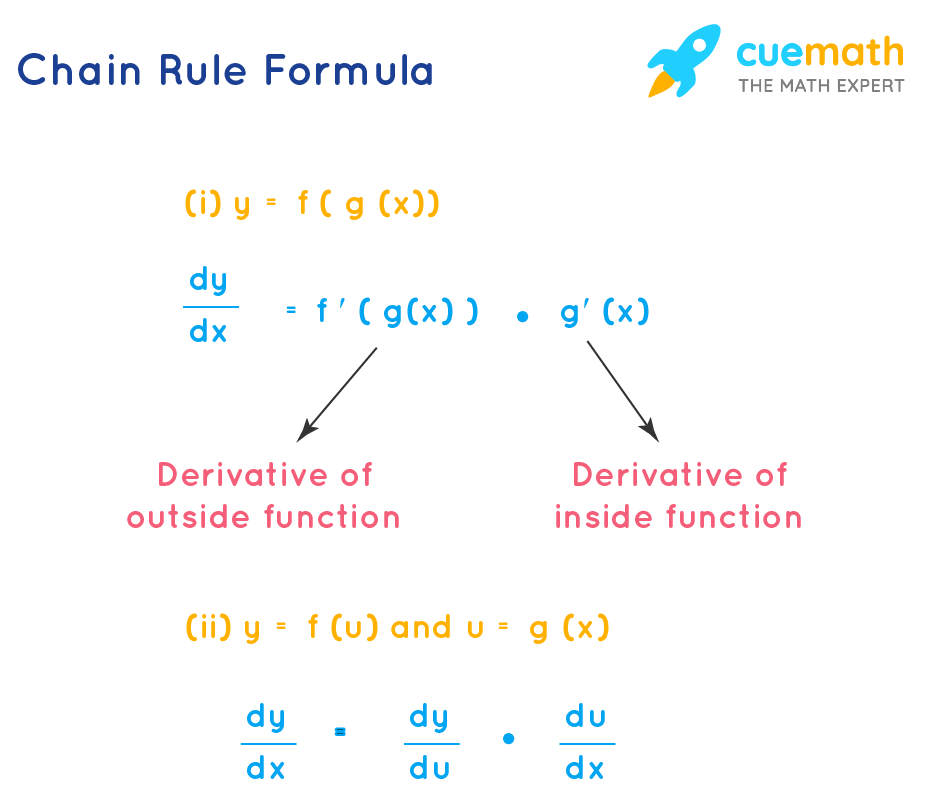
d/dx ( f(g(x) ) = f' (g(x)) · g' (x)

Example : To find the derivative of d/dx (sin 2x), express sin 2x = f(g(x)), where f(x) = sin x and g(x) = 2x.

Then by the chain rule formula,

d/dx (sin 2x) = cos 2x · 2 = 2 cos 2x

Chain Rule Formula 2:

We can assume the expression that is replacing "x" with "u" and applying the chain rule formula.

dy/dx = dy/du · du/dx

Example : To find d/dx (sin 2x), assume that y = sin 2x and 2x = u. Then y = sin u.

By the chain rule formula,

d/dx (sin 2x) = d/du (sin u) · d/dx(2x) = cos u · 2 = 2 cos u = 2 cos 2x

chain rule formula

Chain Rule formula Proof

If y = f(g(x)) = (fog)x, then d/dx ((f(g(x)) = f'(g(x))g'(x)

Proof: Now Δu = g(x+Δx) -g(x)

Therefore Δy/Δx = Δy/ Δu × Δu/Δx

\(\dfrac{f(u+ Δu) - f(u)}{Δu}\) × \(\dfrac{g(x+ Δx) - g(x)}{Δx}\)

As Δx → 0, Δu → 0

Thus lim_Δx→0 Δy/Δx

= lim_Δx→0(Δy/Δu × Δy/Δux)

= lim_Δx→0 \(\dfrac{f(u+ Δu) - f(u)}{Δu}\) × lim_Δx→0 \(\dfrac{g(x+ Δx) - g(x)}{Δx}\)

= f'(u) × u'(x)

= f'(g(x)) g'(x)

Thus lim_Δx→0 Δy/Δx = f'(g(x)) g'(x)

Double Chain Rule

There could be nested functions one over the other, where the functions depend on more than one variable. The chain of smaller derivatives is multiplied together to get the overall derivative. Let there be 3 functions: u, v, w. A function f is a composite of u, v, and w. The chain rule is extended here. If a function is a composition of 3 functions, we apply the chain rule twice. When f = (u o v) o w = df/dx = df/du. du/dv. dv/dw. dw/dx

Example 1: y = (1+ cos 2x)²

y' = 2( 1+ cos 2x) . (-sin 2x). (2)

= - 4(1+ cos 2x) . sin2x

Example 2: y = sin (cos (x²))

y' = cos(cos (x²)). -sin (x²)). 2x

= -2x sin (x²) cos (cos x²)

Note: We do not need to remember the chain rule formula. Instead, we can just apply the derivative formulas (which are in terms of x) and then multiply the result by the derivative of the expression that is replacing x.

For example, d/dx ( (x²+ 1)³) = 3 (x² + 1)² · d/dx (x² + 1) = 3 (x² + 1)² · 2x = 6x (x² + 1)².

Applications of The Chain Rule

This chain rule has broad applications in the fields of physics, chemistry, and engineering. We apply the chain rule:

To find the time rate of change of the pressure,
To calculate the rate of change of distance between two moving objects,
To find the position of an object that is moving to the right and left in a particular interval,
To determine if a function is increasing or decreasing,
To find the rate of change of the average molecular speed,

Let us apply the chain rule to find the equation of the tangent line to the given function y = (5 x⁴ - 2)³at x = 1.

We know that the derivative of the function gives the slope of the line at the given point.

y' = 3 (5 x⁴ - 2) ²× 20 x³

= 60 x³ (5 x⁴ - 2) ²

y' at x =1 gives 60(3)² = 540

We need to evaluate the function and the derivative at the given point
y = ((5 (1)⁴ - 2)³= 3³= 27

Therefore the equation of the tangent line in the slope-intercept form is y = mx+ b ⇒ 27 = 540x + b

We need to find the equation of the tangent line.

Hence substitute (1,27) in the equation of the tangent line, y = 540x + b, we get

27 = 540(1) + b ⇒ b = -513

Thus the equation of the tangent line to the given function y = (5 x⁴ - 2)³applying the chain rule formula is y = 540x - 513

☛ Also Check:

Quotient Rule
Applications of Derivatives
Calculus

Examples Using Chain Rule

Example 1: Find the derivative of y= ln √x using the chain rule.

Solution:

y = ln √x.

f(x) = y is a composition of the functions ln(x) and √x, and therefore we can differentiate it using the chain rule.

Assume that u = √x. Then y = ln u.

By the chain rule formula,

dy/dx = dy/du · du/dx

dy/dx = d/du (ln u) · d/dx (√x)

dy/dx = (1/u) · (1/(2√x))

dy/dx = (1/√x) . (1/(2√x))

dy/dx = 1/(2x) (because u = 1/(2√x)).

y = cos (2x² + 1).

Answer: dy/dx = 1/(2x)
Example 2: A point A is moving along the curve whose equation is y = √(x³+ 56). When A is at (2,8), y is increasing at the rate of 2 units per second. How fast is x changing? (Hint: Use the chain rule.)

To find: dx/dt

Given y = √(x³+ 56) and dy/dt = 2 units / sec

dy/dx = (1/2)(x³+ 56)^- ^1/2(3x²)

=[(3/2) x²] / (x³+ 56)^1/2

Applying the chain rule, dx/dt = dx/dy . dy/dt

Given dy/dx at x = 2

dy/dx at x = 2is [3(4)]/2√64

dy/dx =3/4

dx/dy = 4/3

Thus dx/dt = 4/3 × 2 = 8/3

Answer: x is changing at the rate of 8/3 units per second.
Example 3: Find the derivative of the function y = cos (2x² + 1) using the chain rule.

Solution:

Assume that u = 2x² + 1. Then y = cos u.

By the chain rule formula,

dy/dx = dy/du · du/dx

dy/dx = d/du (cos u) · d/dx (2x² + 1)

dy/dx = - sin u · 4x

dy/dx = -4x sin (2x² + 1) (because u = 2x² + 1).

Answer: The derivative of the given function is, dy/dx = -4x sin (2x² + 1).

go to slidego to slidego to slide

Great learning in high school using simple cues

Indulging in rote learning, you are likely to forget concepts. With Cuemath, you will learn visually and be surprised by the outcomes.

Book a Free Trial Class

Practice Questions on Chain Rule

go to slidego to slide

FAQs on Chain Rule

What Is Chain Rule?

The chain rule is used to find the derivative of a composite function. If there exists a function f of g which in turn is a function of u(x), then the instantaneous change in f with respect to x is given as change in f/ change in x = change in g /change in u × change in u /change in x. If y = f(g(x)), then y' = f'(g(x)). g'(x)

What Is Chain Rule Formula?

The chain rule formula is used to find the derivative of a composite function (i.e, when one function is inside the other). There are two forms of the chain rule formula.

d/dx ( f(g(x) ) = f' (g(x)) · g' (x)
dy/dx = dy/du · du/dx

When To Use Chain Rule Formula?

Usually, all the derivative formulas are in terms of x, for example, d/dx (sin x) = cos x. When x here is replaced with something else here, say, d/dx(sin 3x) =? In such cases, we apply the chain rule formula which says d/dx ( f(g(x) ) = f' (g(x)) · g' (x). Using this, d/dx (sin 3x) = cos 3x · d/dx(3x) = cos 3x (3) = 3 cos 3x.

What Are the Applications of Chain Rule Formula?

The chain rule formula is mainly used to find the derivative of a composite function (a function that is the combination of two or more functions). This chain rule has broad applications in physics, chemistry, and engineering. To find the time rate of change of the pressure, to calculate the rate of change of distance between two moving objects, to find the rate of change of the average molecular speed, we apply the chain rule.

What Is the Difference Between Chain Rule Formula and Product Rule?

The chain rule formula is used to differentiate a composite function (a function where one function is inside the other), for example, ln (x² + 2), whereas the product rule is used to find the derivative of the product of two functions, for example, ln x · (x² + 2).

The chain rule formula is d/dx ( f(g(x) ) = f' (g(x))·g' (x), whereas the product rule formula is d/dx[f(x). g(x)] = f(x) g'(x)+ f'(x) g(x).

What is The Use of Chain Rule?

We use the chain rule in the situations where we require to find the rate of change of a function that is dependent on another function, which is dependent on another function. Here the functions seem to be nested. We use the chain rule formula d/dx ( f(g(x) ) = f' (g(x)) · g' (x) and find the derivative.

How to Use the Chain Rule for Derivatives

Source: https://www.cuemath.com/chain-rule-formula/

Picot Mingat